Research on the practice of massaging the head of a baby with hot water and ointments in Ghana. By Dzamesi Yael (Ph.D. in Science Education)
The hierarchical structure in learning math.
Introduction.
The Hierarchical arrangement of information in the brain was discussed on "Connectionism and hierarchical arrangement" page. The difficulties of the children in Creative Foundation School in learning math was discussed on the pages, "Concluding what was counted in math", "Grouping and separating of numbers", "Interpreting a picture into a math formula", "Addition and subtraction symbols", and "Understanding of quantities". Math is also the most difficult subject in West African Senior School Certificate Examination (WASSCE).
Math is built on hierarchy, and it has a resemblance to the way information moves in the brain. In many schools in Ghana math is taught by rote learning while neglecting the hierarchical structure of the subject. The inability of the pupils in Creative Foundation School to understand the hierarchical structure of math is not a unique case in Ghana. These two aspects of the method of teaching and the capability of children to learn in a certain way affect the way many Ghanaian pupils with poor academic results solve math problem in daily life. Solving simple math problems in a wrong way can be not only because of method of teaching but also as a result of defect or injury in the hierarchical structure of the nodes in the neural system of the brain. Therefore, it is worthwhile contribution to understand the hierarchical structure of math.
For illustration, let us assume for instance that each mathematical operation or mathematical concept is represented in the brain by one certain neural population. How many populations of nerve cells and synapses are joining to express or store one single concept? As the concept is simpler, it needs less nerve cells and synapses to express or store it. The concept of addition is the simplest, so it involves less nerve cells and synapses. Followed in complexity of neural structure is subtraction, multiplication and the last is division. Since conceptual understanding of multiplication is based on addition, these 2 neural populations that express the concepts must be connected to each other by other neurons and synapses. Division is based on multiplication, subtraction and addition, and therefore, this concept involves 4 neural populations that are connected between them. Therefore, as the mathematical concept is more complex it involves the activation of more neural populations. This set of mathematical conceptual understanding is based on hierarchical path of reasoning, which must be supported by the ability of the neurons to build a complicated neural network of communication in stages of a hierarchical structure or order.
An injury to the head can disturb and change the way the nerve cells are connected to each other, therefore, the hierarchical path that would allow the person to think in reasonable way would be disrupted. The hot water and the Robb ointment that are applied on the head of a baby in the critical period of forming the basic neural infrastructure for the future expansion of the neural network, can affect and damage the formation of the neural infrastructure. Therefore, it will be difficult for the children who passed the hot water to build that kind of complicated hierarchical neural network and understand the hierarchical structure of math, meaning, that each math operation is based on another simpler operation.
Math as a hierarchical path of reasoning.
The following Figures 1,2 3, and 4, explain the hierarchical structure of math and the difficulty the children had in learning it.
In order to achieve the correct neural network for the meaningful understanding of the four basic mathematical operations, the method of teaching should help to store the information in the hierarchical arrangement. Figure 1 describes the stages of teaching that is based on the hierarchical arrangement whereby complicated concepts are being taught after the understanding of simpler ones. However, in many Ghanaian schools the method of reciting shown in Figure 2, is common, and therefore no conceptual understanding can be achieved.
Figure 1: The hierarchical structure of the four basic mathematical operations

Figure 2: The common reciting way of learning the three basic mathematical operations in most of Ghanaian schools.
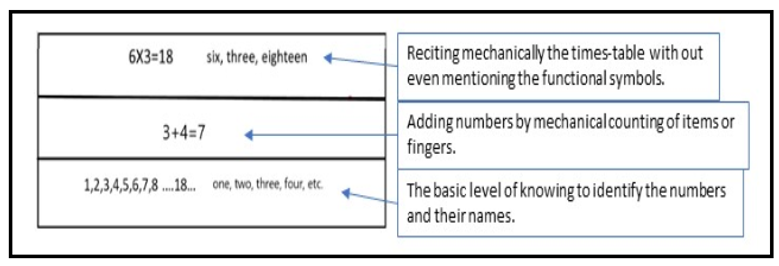
No conceptual understanding or meaningful learning is achieved in the reciting way shown in Figure 2.
In the light of the potential damage to the neural network of the brain of a baby because of the use of the hot water and the Robb ointment, it is possible that this is the only way the pupils can retain the three basic mathematical operations, even though, not necessarily they will be able to use all of them. Among many pupils that could not pass the Basic Education Certificate Examination (BECE) that is needed for the entrance to the secondary school because of their low academical results, it is common to see that they are not able to perform subtraction or more complicate addition in a correct way. Figure 3 describes subtraction of numbers with upper digit that is smaller than the down. Figure 4 describes complicated addition involving numbers on the 'ones' column (right side) that their sum is above 10, .
Figure 3: The local way of solving Figure 4: The local way of solving
difficult subtraction. difficult addition.
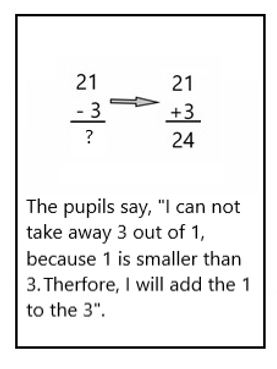
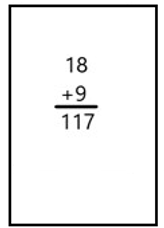
In both examples (Figures 3 and 4) there is no understanding of the concepts of 'ones' and 'tens'. These two concepts were difficult to grasp by the children of Primary 1 and 2 in Creative Foundation School. We used sets of 10 matches tied together with elastic band as a representative of the concept of 'tens' and single matches to represent the concept of 'ones'. However, the children continually forgot that there are 10 matches in the bundle of the 'tens', even though they opened the bundle, counted the matches and tied them together many times.
The market women in Ghana.
Most market women can not add two numbers that their sum is above 10 without the aid of the calculator. For many of them the only operation they can do on the calculator is addition even in a case that somebody buys more than one of the same items. For example, tomatoes in a basket cost ₵GH 12. If a customer buys three baskets of tomatoes the seller will press three times the sum of 12 and the symbol of (+) on the calculator to get the amount of money the customer shall pay, which is ₵GH36. Sellers with experience know how to subtract the amount due for payment from a note of money which is ₵GH 50 by heart (It is not clear if it is done by memorizing figures or by actual calculations). However, new sellers in the market, the girls at the age of Junior Secondary School, make mistakes in their calculations by heart. It is possible that the illiterate women in the markets have better conceptual understanding of quantities, addition, and subtraction than many pupils since they deal with the practical aspect of these operation every day.
Learning and neural connections.
The way of learning a topic has a great effect on how the information can be organized in the long-term memory. As demonstrated here, the reciting way of learning can not help in this task. The brain is not a passive storage whereby information is only kept there. It is a dynamic storage that has to continuously changing its storage of information according to the needs. The process of learning involves getting rid from information that is no longer correct or relevant and taping from the long-term memory relevant information to the situation or issue to be solve. For example, getting rid from an old wrong way of solving subtraction formula as is shown in Figure 3 and taping from the existing information the counting order in order to learn the difference between the concept of 'ones' and 'tens' and to integrate these new understanding with the existing ones. The working tool that helps to do all these is called working memory. The working memory serves as an active sieve in the process of learning new information. The working memory taps certain information from the vast information in the long-term memory. Learning therefore is a continual change in the neural connection, building new connections and destroying unnecessarily connections between neurons.
Since the hot water massage to the head of a baby can affect the infrastructure of the neural network, it would affect the complexity and the organization of the long-term memory neural network, and the efficiency of the working memory. This will lead to impaired learning capabilities as it found among Ghanaian pupils in Ghana.